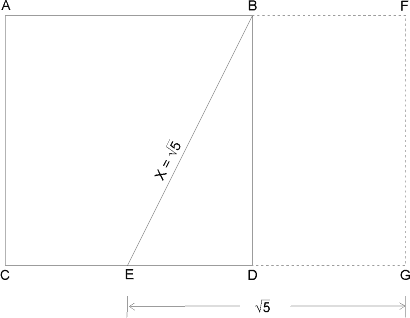
enviroklion.blogg.se - Fibonacci rectangle

FIBONACCI RECTANGLE SERIES

Of the sines, cosines and tangents of simple angles like 36° and 54°.

An auxiliary page on Exact Trig Values for SimpleĪngles explores the many places that Phi and phi occur when we try to find the exact values.

Unexpected places that the golden section (Phi) occurs in Geometry and in Trigonometry:Īnd Penrose Tilings where we phind phi phrequently! Two more pages look at its applications in Geometry:įirst in flat (or two dimensional) geometry and then in The golden section is also called the golden ratio, the golden mean and Phi. It about the occurrence in nature of the golden section and It is an excellent introduction to the golden section. Phi and phi have some interesting and unique properties such as 1/phiīroadcast on 13 March 2002 on BBC Radio 4 (with a small p), the Greek letter φ here. with exactly the same decimal fraction part as Phi. The other number also called the golden section Which we call Phi (note the capital P), the Greek letter Φ, on these pages. What happens if we use the Fibonacci numbers as the column headers? We use base 10 (decimal) for written numbers but computers use base 2 (binary). Involve Phi and phi - the golden section numbers.įibonacci bases and other ways of representing integers Yes there is! This page shows several and why they Is there a direct formula to compute Fib(n) just from n? Prime Fibonacci numbers are identified up to Fib(500). We start with a completeĬompletely factorized up to Fib(300) and all the The following pages give you lots of opportunities toįind your own patterns in the Fibonacci numbers. Try out the simple Fibonacci numbers trick! If you want to look like a number wizard to your friends then To generate all right-angled triangles with integers sides based on Pythagoras Theorem.

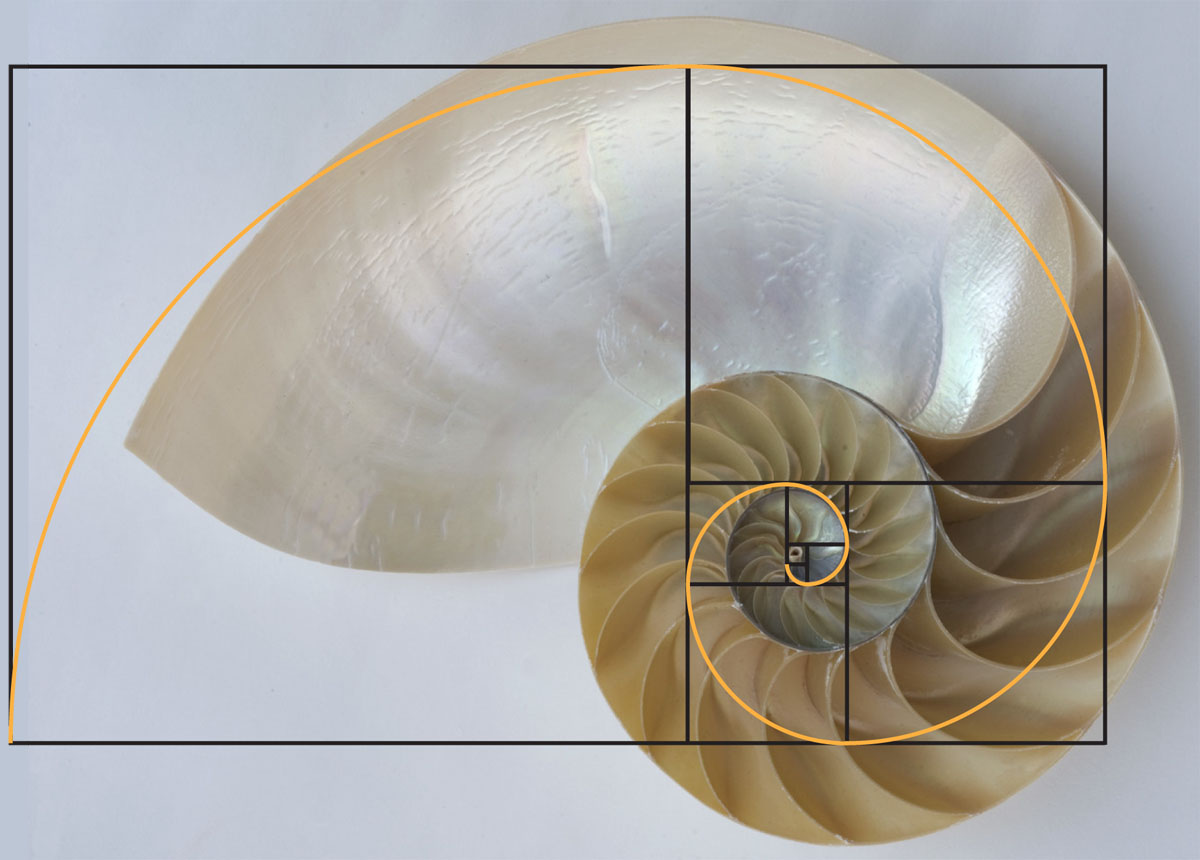
FIBONACCI RECTANGLE SERIES

The Fibonacci numbers in Pascal's Triangle using the Fibonacci series Looks at the patterns in the Fibonacci numbers themselves: The Mathematical Magic of the Fibonacci numbers.The golden section numbers are also written using the Greek letters Phi

The Intriguing Mathematical World of Fibonacci and Phi Real puzzle - can you supply a simple reason why? The Fibonacci numbers occur - and that's the Puzzles, I do not know of any simple explanations of why Pieces makes an additional square appear,ĭid you know the same puzzle can be rearranged to make aĭifferent shape where a square now disappears? If you know the Fibonacci Jigsaw puzzle where rearranging the 4 wedge-shaped The Fibonacci numbers are the answers - well, all but ONE, but WHICH one? With electrical resistance and lots more puzzles Giving change and a game with match sticks and even Has the Fibonacci numbers in brick wall patterns,įibonacci bee lines, seating people in a row and the Fibonacci numbers More.Ī pair of pages with plenty of playful problems to perplex the Introduction to Continued Fractions An unusual method of writing fractions that has many advantages.Īn Exact Fractions Calculator and converter Fractions to and from Decimal, Continued Fractions from and to Fractions withĪn expression evaluator and many in-built functions and all to as many decimal places as you like!. More on Runsums Integer Sums or Partitions of an integer The number 2016 The number 2021 Fractions Fractions and Decimals - their periods and patterns and in non-decimal bases.įarey Fractions and Stern-Brocot Tree Calculators Two ways of arranging all fractionsĮgyptian fractions The Egyptians only had unit fractions of the form 1/n. Runsums Numbers which are the sum of a run of consecutive whole numbers More on Polygonal Numbers Central polygonal numbers, matchstick figures, 3D solid shapes and higher dimensions Integer Palindromes Polygonal and Figurate Numbers Triangular, Square, Pentagonal.

Primes & Factors Calculator Integer Bases many kinds of bases to represent integeres Introduction to trilinear and barycentric coordinates, links to Clark Kimberling'sĮncyclopedia of Triangle Centers (ETC) with automatic lookup 3, 4, 5.Įxact Trig Values for Simple Angles Which angles have a simple exact value for their sine,cosine or tangent?Ī Triangle Convertor for Cartesian, Trilinear and Barycentric Coordinates Pythagorean triangles Right-angled triangles with integer sides, e.g. Other Maths Pages at this site: Triangles and Geometry